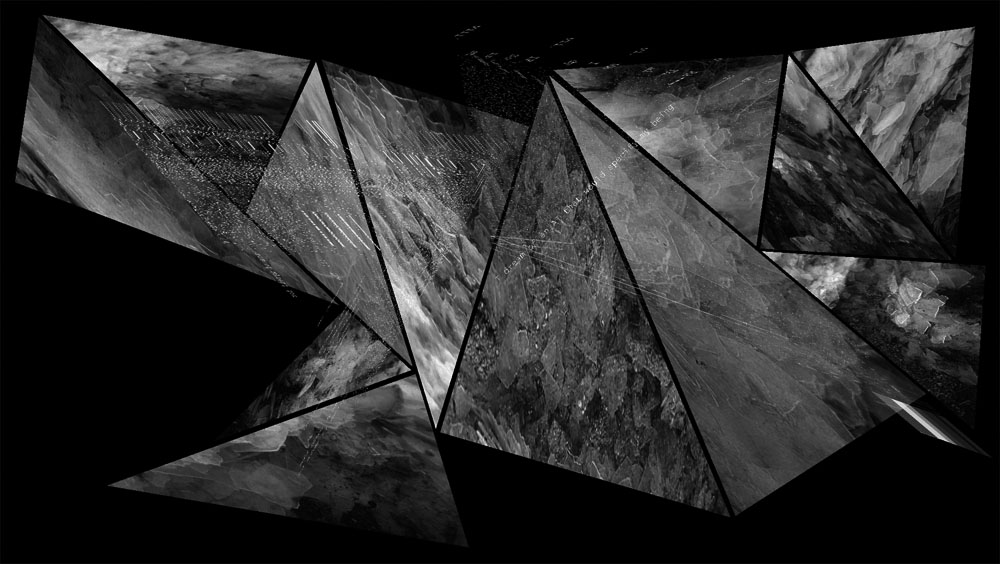
„lisa_GI“, 4th grade glicth, 460×429, original photo courtesy of Lisa Gi, 2013
i = i + 1
fake UFO art
little red riding hood
„the code is cold“ – manifesto
Leading up to my solo exhibition in autumn I am glad to introduce the website that will document the process of setting up the complex network of real and virtual art that is „the code is cold“.
5 Degrees Of Separation – Premiere @ Pottporus Festival

5 Degrees Of Separation by KooneCompany
Weitere Bilder und Infos zur Premiere hier >>
„As KooneCompany was chosen by the Goethe Institut Montreal for their Dance Residency Programm in summer 2011, we created the 1 hour length piece: „5 Degrees Of Separation“ .
After the good work in Montreal we take the next step and show the premiere of the piece on the 3rd of november in Herne at the Kulturzentrum Herne… The premiere will be part of the well known „Pottporus Festival“ initiated by Pottporus e.V. / Renegade Theatre .“
(from http://koonecompany.blogspot.de/2011/10/5-degrees-of-separation-premiere.html)
Und hier das ursprüngliche Konzept von uns >>
code poetry
found:
RANKX-Funktion (DAX): RANKX(<table>, <expression>[, <value>[, <order>[, <ties>]]])
--->
Proving rank(℘(x))=rank(x)+
Enderton defines the rank of a set A to be the least ordinal α such that A⊆Vα (equivalently, A∈Vα+). He the derives the following identity: rank(A)=⋃{(rank(x))+:x∈A} for all sets A.
In Exercise 30 of Chapter 7, the reader is asked to prove several identities involving rank. For instance, that rank{a,b}=max(rank(a),rank(b))+. I am having trouble proving the second identity, that rank(℘(x))=rank(x)+ for all sets x. I am not sure whether I am missing some elementary identity that would let me prove the identity, or whether I am misunderstanding the definition of rank.
Clearly, z∈rank(℘(x))⇔(∃y)(y⊆x∧(z∈rank(y)∨z=rank(y)). On the other hand z∈(rank(x))+⇔(∃y)(y∈x∧z∈(rank(y))+)∨z=rank(x). I'm not sure why the first statement should imply the second, and conversely, however.
>> elementary-set-theory
--->
Now isn’t that poetic…
this I call home – glitch art
post privacy art #2
„before the fuck“, social glitch, 4000x3000px, 2012-2013
Sometimes, no need for glitching, society glitches all by itself. Some call this post privacy art…
„before the fuck“ is an ongoing series of digital stills taken from porn sites and chat pages in that particular moment, when the user / actor leaves the room and us with that distinctive feeling of loneliness and bleak despair. It is a look inside the sadness and harshness of modern day sexuality when confronted with ‚the market‘.
post privacy art #1
„m4usism4us“, video-art / installation [pd/GEM], 2011
The video installation „m4usism4us“ is about ones image & self definition in the realm of interactive mass media. It is based on a PureData [pd] / GEM installation and is slightly retreated.
Tnx 2 m4usism4us 4 being herself
music: MK_ultra
ARTE about post privacy art >>